AP Statistics
Sections: 1.|  Inference for a Population Proportion  2.| Comparing Two Proportions

   Comparing Two Proportions

There's really nothing new to learn to compare two proportions because we know how to compare means. Proportions are just means! The proportion having a particular characteristic is the number of individuals with the characteristic divided by total number of individuals. Suppose we create a variable that equals 1 if the subject has the characteristic and 0 if not. The proportion of individuals with the characteristic is the mean of this variable because the sum of these 0s and 1s is the number of individuals with the characteristic.

The Sampling Distribution of

Both are random variables, that is, if we took repeated samples of the same size their values would vary. The statistic, , is the difference between these two random variables. Earlier we saw that if X and Y are independent random variables,

Using these facts gives us some important information about the sampling distribution of :

The mean of is

The difference of the sample proportions is an unbiased estimator of the difference of the population proportions.

The variance of is

provided the sample proportions are independent. Remember that variances add but standard deviations do not.

When the samples are large, the distribution of is approximately normal.

The figure below illustrates the distribution of .

Select independent SRSs from two populations having proportions of successes p1 and p2. The proportions of successes in the two samples are . When samples are large, the sampling distribution of the difference is approximately normal.

Confidence Intervals

The standard deviation of is the square root of the variance:

To obtain a confidence interval replace the population proportions p1 and p2 in the expression by the sample proportions. the result is the standard error of the statistic :

The formula for the confidence interval has the form  estimate ± z*SEestimate

 

Confidence Intervals for Comparing Two Proportions

An approximate confidence interval for the difference between two population proportions (p1-p2) based on two independent samples of size n1 and n2 with sample proportions and is given by

where z* is the upper (1 -C)/2 standard normal critical value. The formula is used when the populations are at least 10 times larger than the samples and

are greater than or equal to 5.

Even though this looks different from other formulas we've seen, it's nearly identical to the formula for the "equal variances not assumed" version of Student's t test for independent samples. The only difference is that the standard deviations are calculated with n in the denominator instead of n-1.

Example:

Suppose that in a sample of 68 urban students, 42 have had a flu shot and in a sample of 65 rural students, 30 have had a flu shot. The two sample proportions are 0.800 and 0.462. 

Data summary: n1 = 68,  = 0.618,  n2 = 65, and = 0.462

Check assumptions: the smallest value of is 26(1 - 0.618) which equals 9.932. The confidence intervals based on normal sampling distributions are valid. There is one caution here, we don't know if the samples are SRSs. We can proceed with building the confidence interval but we must be cautious about drawing conclusions based on the interval.

A 95% confidence interval for the difference in population proportions is

= 0.156 ± 0.167 = (-0.011, .323)

We can be 95% confident that the proportion of urban students with flu shots is between -1.1% and 32.3 different than the proportion of rural students with flu shots. This confidence interval is fairly wide because the sample sizes are small.

Try Self Check 18

Significance Tests

We want to investigate the differences between two sample proportions from two distinct populations. Is there a true difference in the proportions or is the difference due to chance.

To test the null hypothesis H0: p1 = p2 against a one- or two-sided alternative hypothesis Ha, first compute a pooled estimate for the parameter,

 

where X1 and X2 represent the number of "successes" in each population sample. This estimate for a single sample proportion agrees with the null hypothesis, where the two proportions are assumed to be equal. Calculate the pooled standard error SE, which is equal to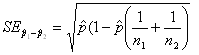

The test statistic z =  /SE  follows the standard normal distribution (with mean = 0 and standard deviation = 1). The test statistic z is used to compute the p-value for the standard normal distribution, the probability that a value at least as extreme as the test statistic would be observed under the null hypothesis. Given the null hypothesis that the population proportions are equal, the p-values for testing H0 against each of the possible alternative hypotheses are:

P(Z > z) for Ha: p1 > p2        
P(Z < z) for Ha: p1 < p2        
2P(Z>|z|) for Ha: p1 p   .

Example:

Students in grades 4-6 were asked whether good grades, athletic ability, or popularity was most important to them. Is popularity more important to girls or boys?

169 girls and 166 boys were included in the survey. Of the girls, 58 ranked popularity most important, compared to 40 of the boys.

The sample proportion for girls is 58/169= 0.343, and for boys is 40/166 = 0.237.

Null hypothesis                  H0: p1 = p2

Alternative hypothesis        Ha: p1 ≠ p2

To test the difference of the proportions of girls and boys who rated popularity most important, first compute the pooled estimate

Using this value we need to check our conditions to see if a 2-sample z procedure is valid.

All are clearly greater than 5 so we are safe to use the two-sample z procedure.

Calculate the standard error using

SE = 0.0497

The test statistic is

Since this is a two-sided hypothesis, we are interested in the probability 2P(Z > 2.05) = 2(1 - P(Z < 2.05)) = 2(1 - 0.9798) = 2(0.0202) = 0.0404.

Conclusion: Since the p-value is small it may be safe to say that girls in grades 4-6 find popularity to be more important than boys in grades 4-6. Notice that the finding is significant at the 0.05 level, although it is not significant at the 0.01 level.

Try Self Check 19

Below is a flow chart that will help you choose the correct procedures when doing inferences on means and proportions. You are not allowed to use this chart on the AP Statistics exam but studying it will help understand which procedure to use.

   

Proceed to Statistics Assignment 11: Working with Two Proportions

Proceed to the Unit 5 Exam Objective Questions and Free Response (The Objective Questions contain 8 Multiple Choice and 8 True/False.)
 
© 2004 Aventa Learning. All rights reserved.