Try It
Proving Lines Parallel Practice
Practice Problem #1
What must x equal for these lines to be parallel?
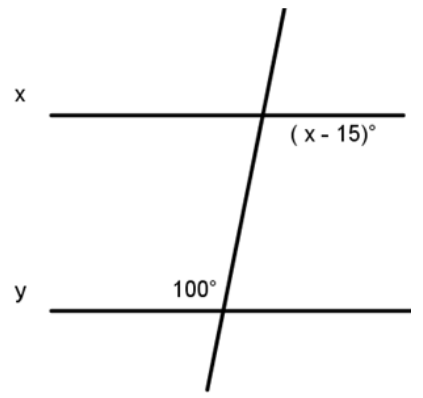
What is the relationship between thse angles? Answer: alternate interior
Because they are on opposite sides of the transversal, they are alternate. Because they are contained between the two parallel lines, they are interior. So they are alternate interior angles.
Now that we know these are alternate interior angles, what is the relationship between these angles? Are they congruent, complementary, or supplementary? Answer: congruent
Alternate interior angles are congruent. Which equation should we use to solve for x?
x - 15 +100 = 90
x - 15 = 100
x - 15 + 100 = 180
Answer: x - 15 = 100
Now we just solve:
x - 15 = 100
x = 115
Practice Problem #2
What must x equal for these lines to be parallel?
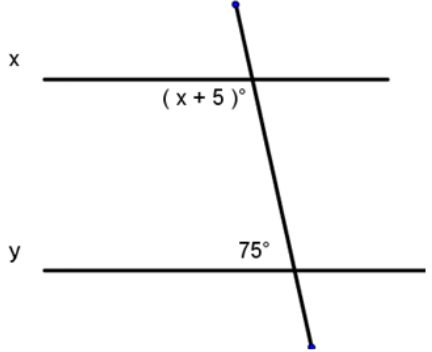
What is the relationship between thse angles? Answer: same side interior
Because they are on the same side of the transversal, they are same side. Because they are contained between the two parallel lines, they are interior. So they are same side interior angles.
Now that we know these are same side interior angles, what is the relationship between these angles? Are they congruent, complementary, or supplementary? Answer: supplementary
Alternate interior angles are supplementary. Which equation should we use to solve for x?
x - 15 +75 = 90
x - 5 = 75
x + 5 + 75 = 180
Answer: x + 5 + 75 = 180
Since the angles are supplementary, and them together and set the sum equal to 180.
Now we just solve:
x - 5 + 75 = 180
x + 80 = 180
x = 100
Practice Problem #3
What must x equal for these lines to be parallel?
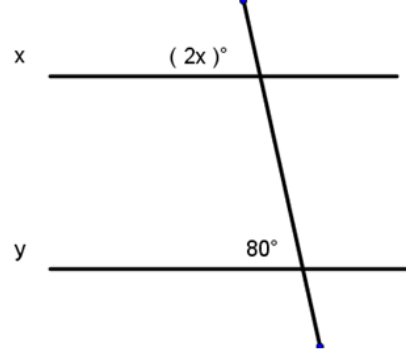
What is the relationship between thse angles? Answer: corresponding
Because they are on the same place in each set of angles, they are corresponding.
Now that we know these are corresponding angles, what is the relationship between these angles? Are they congruent, complementary, or supplementary? Answer: congruent
Alternate interior angles are congruent. Which equation should we use to solve for x?
2x + 80 = 90
2x = 80
2x + 80 = 180
Answer: 2x = 80
Since the angles are congruent, set them equal to one another to solve for x.
Now we just solve:
2x = 80
x = 40
Practice Problem #4
What is the relationship between these angles?
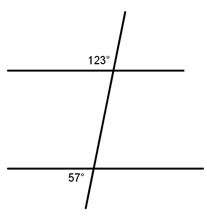
Answer: same side exterior
Practice Problem #5
What is the relationship between these angles?
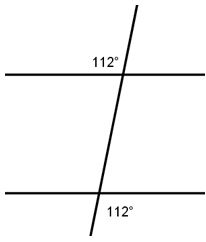
Answer: alternate exterior
Practice Problem #6
What is the relationship between these angles?
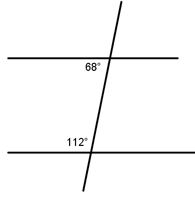
Answer: same side interior
Practice Problem #7
What is the relationship between these angles?
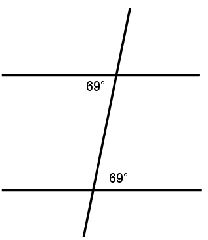
Answer: alternate interior
Practice Problem #8
What is the relationship between these angles?
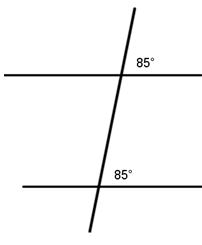
Answer: corresponding