Try It
Parallel Lines Practice
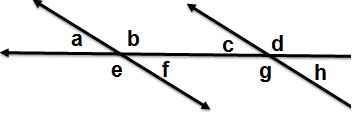
Questions 1 -3 refer to this image.
- Which angle forms an alternate exterior angle pair with angle b?
- d
- f
- g
- e
Answer: c. The correct answer is g.
Angles b and g are alternate exterior angles because the angles are outside the parallel lines, and on opposite sides of the transversal. Angles a and h form the other alternate exterior angle pair in this diagram. - Angle h corresponds to angle _____.
- g
- a
- d
- f
Answer: c. The correct answer is d.
Other corresponding angle pairs in the diagram are: a and e; b and f; c and g. - Which angle forms a same side interior angle pair with angle e?
- c
- b
- h
- e
Answer: a. The correct answer is c.
Angles c and e form a same side interior angle pair because they are inside the parallel lines and on the same side of the transversal. Angles d and f form the other same side interior angle pair in this diagram. - Use the diagram to identify the pair of angles e and h.
- same side exterior
- same side interior
- alternate interior
- alternate exterior
Answer: a. same side exterior
- Use the diagram to identify the pair of angles f and h.
- corresponding
- same side interior
- alternate interior
- alternate exterior
Answer: a. corresponding
- Use the diagram to identify the pair of angles b and g.
- corresponding
- same side exterior
- alternate interior
- alternate exterior
Answer: c. alternate interior
- __________ are created where a transversal crosses two (usually parallel) lines. Each pair of these angles are inside the parallel lines, and opposite sides of the transversal.
- Same side interior
- Alternate exterior
- Alternate interior
- Same side exterior
Answer: c. Alternate interior
- Angle pair created where a transversal crosses two (usually parallel) lines. Each pair of these angles are outside the parallel lines, and on the same side of the transversal.
- same side interior angles
- same side exterior angles
- alternate interior angle
- alternate exterior angle
Answer: d. alternate exterior angle
- Angle pair created where a transversal crosses other (usually parallel) lines. These angles are the ones at the same location at each intersection.
- corresponding
- alternate exterior
- alternate interior
- vertical
Answer: a. corresponding
- Angle pair created where a transversal crosses two (usually parallel) lines. Each pair of these angles are outside the parallel lines, and on opposite sides of the transversal.
- same side interior
- same side exterior
- alternate interior angles
- alternate exterior angles
Answer: d. alternate exterior angles
- Angle pair created where a transversal crosses two (usually parallel) lines. Each pair of these angles are inside the parallel lines, and on the same side of the transversal.
- same side interior
- same side exterior
- alternate interior
- corresponding
Answer: a. same side interior
- Alternate exterior angles, alternate interior angles, vertical angles, and corresponding angles all have what type of angle relationships?
- congruent
- supplementary
Answer: a. congruent
- Same side interior, same side exterior, and linear pair angles all have what type of angle relationships?
- congruent
- supplementary
Answer: b. supplementary
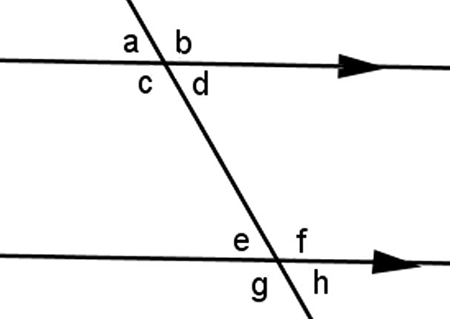
Questions 4 - 6 refer to this image.